What is Algebra?

Algebra – branch of mathematics in which symbols are used to represent

                numbers and quantities in equations and formulas, and which deals with

                the study of rules of operations and relations, and the constructions and concepts

                arising from them.

             - the mathematics of generalized arithmetical operations

             - the branch of mathematics concerning the study of structure, relation and quantity

             - came from the Arabic words “al jabr” (al – the, jabr- reuniting what is broken)

               which literally means “restoration”

Algebra is great fun - you get to solve puzzles!

A Puzzle

What is the missing number?

-

2

=

4

OK, the answer is 6, right? Because 6 - 2 = 4. Easy stuff.

Well, in Algebra we don't use blank boxes, we use a letter (usually an x or y, but any letter is fine). So we would write:

x

-

2

=

4

It is really that simple. The letter (in this case an x) just means "we don't know this yet", and is often called theunknown or the variable.

And when you solve it you write:

x

=

6

Why Use a Letter?

	Because:
	it is easier to write "x" than drawing empty boxes (and easier to say "x" than "the empty box").
	if there were several empty boxes (several "unknowns") we can use a different letter for each one.

So x is simply better than having an empty box. We aren't trying to make words with it!

And it doesn't have to be x, it could be y or w ... or any letter or symbol you like.

How to Solve

Algebra is just like a puzzle where you start with something like "x-2 = 4" and you want to end up with something like "x = 6".

But instead of saying "obviously x=6", use this neat step-by-step approach:

• Work out what to remove to get "x = ..."
• Remove it by doing the opposite (adding is the opposite of subtracting)
• Do that to both sides

Here is an example:

We want to remove the "-2"	To remove it, do the opposite, in this case add 2:	Do it to both sides:	Which is ...	Solved!

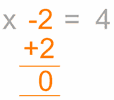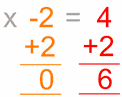Why did we add 2 to both sides?

To "keep the balance"...

	Add 2 to Left Side	Add 2 to Right Side Also
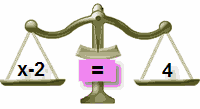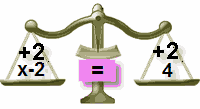In Balance	Out of Balance!	In Balance Again

Just remember this:

To keep the balance, what you do to one side of the "=" you should also do to the other side!

Another Puzzle

Solve this one:

x

+

5

=

12

Start with:	x + 5 = 12
What you are aiming for is an answer like "x = ...", and the plus 5 is in the way of that! If you subtract 5 you can cancel out the plus 5 (because 5-5=0)
So, let us have a go at subtracting 5 from both sides:	x+5 -5 = 12 -5
A little arithmetic (5-5 = 0 and 12-5 = 7) becomes:	x+0 = 7
Which is just:	x = 7
	Solved!
(Quick Check: 7+5=12)

(c)www.mathsisfun.com