Parallel and Perpendicular Lines
How to use Algebra to find parallel and perpendicular lines.
Coordinates
I will be using Cartesian Coordinates, where you mark a point on a graph by how far along and how far up it is.
| |
| Example: The point (12,5) is 12 units along, and 5 units up. |
You should also know about the equation of a line:
y = mx + b
|
Parallel Lines
How do you know if two lines are parallel?
Their slopes are the same!
|
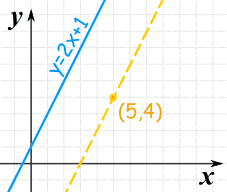
Example:
The slope of y=2x+1 is: 2
The parallel line must have the same slope!
Let us put that in the "point-slope" equation of a line:
y - y1 = 2(x - x1)
And now put in the point (5,4):
y - 4 = 2(x - 5)
And that is a good answer!
But let's also put it in the "slope-intercept (y = mx + b)" form:
y - 4 = 2x - 10
y = 2x - 6
|
Vertical Lines
Be careful! They may be the same line (just with a different equation), and so would not really be parallel.
How to know if they are really the same line? Check their y-intercepts.
But this does not work for vertical lines ... I explain why at the end
Not The Same Line
Example: is y=3x+2 parallel to y-2=3x ?
For y=3x+2: the slope is 3, and y-intercept is 2
For y-2=3x: the slope is 3, and y-intercept is 2
In fact they are the same line and so are not parallel
|
Perpendicular Lines
Two lines are Perpendicular if they meet at a right angle (90°).
How do you know if two lines are perpendicular?
When you multiply their slopes, you get -1
This will show you what I mean:
When you multiply their slopes, you get -1
 |
These two lines are perpendicular:
If we multiply the two slopes we get:
2 × (-0.5) = -1
|
Using It
OK, if we call the two slopes m1 and m2 then we could write:
m1m2 = -1
Which could also be:
| m1 = -1/m2 | or | m2 = -1/m1 |
So, to go from a slope to its perpendicular:
- calculate 1/slope (the reciprocal)
- and then the negative of that
In other words the negative of the reciprocal.
Example:
Find the equation of the line that is
- perpendicular to y = -4x + 10
- and passes though the point (7,2)
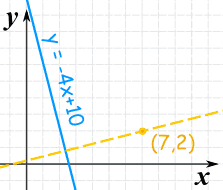
The slope of y=-4x+10 is: -4
The negative reciprocal of that slope is:
m = - 1 = 1 -4 4
So the perpendicular line will have a slope of 1/4:
y - y1 = (1/4)(x - x1)
And now put in the point (7,2):
y - 2 = (1/4)(x - 7)
And that is a good answer!
But let's also put it in "y=mx+b" form:
y - 2 = x/4 - 7/4
y = x/4 + 1/4
Find the equation of the line that is
|  |
The slope of y=-4x+10 is: -4
The negative reciprocal of that slope is:
| m = - | 1 | = | 1 |
| -4 | 4 |
So the perpendicular line will have a slope of 1/4:
y - y1 = (1/4)(x - x1)
And now put in the point (7,2):
y - 2 = (1/4)(x - 7)
And that is a good answer!
But let's also put it in "y=mx+b" form:
y - 2 = x/4 - 7/4
y = x/4 + 1/4
Vertical Lines
The previous methods work nicely except for one particular case: a vertical line:
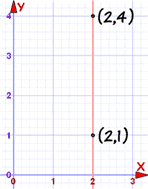 |
In that case the gradient is undefined (because you cannot divide by 0):
|
So just rely on the fact that:
- a vertical line is parallel to another vertical line.
- a vertical line is perpendicular to a horizontal line (and vice versa).
(c)http://www.mathsisfun.com